Answer:
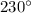
Explanation:
For convenience, I labeled some points as shown in the attached picture.
Also, I assume
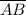 and
and
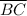 are tangents to the circle.
are tangents to the circle.
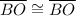 (reflexive property)
(reflexive property)
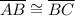 (tangents drawn from a common external point are congruent)
(tangents drawn from a common external point are congruent)
 (right angles are congruent)
(right angles are congruent)
Therefore, we know
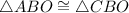 by HL.
by HL.
Thus, by CPCTC,
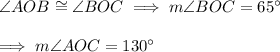
This means the measure of minor arc AC is
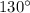 , and thus
, and thus
