Answer:

Explanation:
Given polynomial function :-
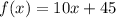
To find :-

Solution:-

Here, the value of x is 7(given).
So, Substitute the value of x on the RHS and on LHS :-
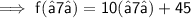
Simplify the RHS:-
Multiply 10 and 7 :-
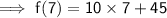
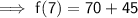
Add 70 and 45 :-
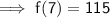
We're done!
Hence, the value of f(7) would be,
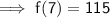

I hope this helps!
Let me know if you have any questions.