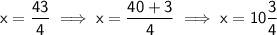Answer:
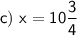
Explanation:
Given equation:

Step 1: Convert the mixed numbers into improper fractions.
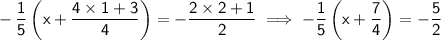
Step 2: Distribute -⅕ through the parentheses.
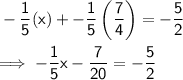
Step 3: Rewrite the equation with a common denominator of 20.

Step 4: Multiply both sides by 20.

Step 5: Add 7 to both sides.
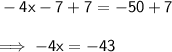
Step 6: Divide both sides by -4.

Step 7: Convert the answer back into a mixed number.