Question

sketch a unit circle and label the terminal points corresponding to theta = pi/3, 2pi/3, 4pi/3, 5pi/3 Include the coordinates of each point

Answer 1

Answer: The figure is attached herewith.

Step-by-step explanation: We are given to sketch a unit circle and label the terminal points corresponding to

`\theta=(\pi)/(3),~2(\pi)/(3),~3(\pi)/(3),~4(\pi)/(3),~5(\pi)/(3).\\\\\\\Rightarrow \theta=60^\circ,12^\circ,180^\circ,240^\circ,300^\circ.`

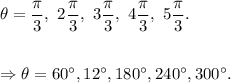
In the attached figure, we have drawn a unit circle, with centre at O(0,0) and radius 1 unit. We have labelled the points A, B, C, D and E corresponding to the angles below

∠AOX = 60°,

∠BOX = 120°,

∠COX = 180°,

∠DOX = 240°,

∠EOX = 300°.

And the coordinates are

[tex]A(\dfrac{1}{2},\dfrac{\sqrt 3}{2}),~B(-\dfrac{1}{2},sqrt 3), C(-1,0), D(-\dfrac{1}{2},-sqrt 3) and E(\dfrac{1}{{2},-\sqrt 3).

Thus, the answer is complete.