Answer:
The range of the function is: -∞≤y≤∞.
Explanation:
Consider the provided function
![y=\sqrt[3]{x+8}](https://img.qammunity.org/2020/formulas/mathematics/college/nisyshqe1m2mc2hciw061b97ddpm8ddyy1.png)
The range of the function is the set of all values which a function can produce or the set of y values which a function can produce after substitute the possible values of x.
The range of a cubic root function is all real numbers.
Now consider the provided function.
![y=\sqrt[3]{x+8}](https://img.qammunity.org/2020/formulas/mathematics/college/nisyshqe1m2mc2hciw061b97ddpm8ddyy1.png)
The above function can be written as:

Taking cube on both sides.
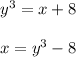
The graph of the function is shown in figure 1:
For any value of x we can find different value of y.
Here, the cube root function can process negative values. Since, the function can produce any values, the range of the given function is -∞≤y≤∞ .
Therefore, the range of the function is: -∞≤y≤∞.