Question

A tanks length is 3 feet longer than its width. The height is 1 foot less than the width. The volume of the tank is 160 cubic feet. What is the width of the tank?

Answer 1

The width of the tank is 5 feet.

Explanation:

Let the width of the tank be
`w ft`.

Then, the length of the tank will be
`l=(w+3)ft`

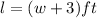
and the heigth will be
`h=(w-1)ft`.

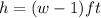It was given that the volume of the tank is
`160ft^3`.

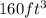Recall that the tank is a cuboid or a rectangular prism.

The volume is given by;

`V=l*b * w * h`.

We substitute the given values into the formula to obtain;

`160=(w+3)(w)(w-1)`

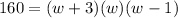
We expand to obtain

`160=w(w^2-w+3w-3)`

`160=w(w^2+2w-3)`

`160=w^3+2w^2-3w`

`w^3+2w^2-3w-160=0`

`\Rightarrow w=5`

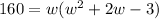
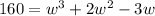
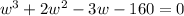
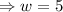
Therefore the width of the tank is 5 feet.