Answer:
3081.24 cm³ (nearest hundredth)
Explanation:
Formulas
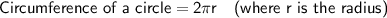

If a rectangular piece of paper is rolled along its length to form a cylinder:
- circumference = 44 cm
- height = 20 cm
To calculate the volume of the formed cylinder, determine the radius by using the circumference formula:
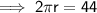
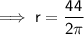
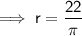
Substitute the round radius into the formula for Volume and solve for V:
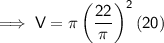

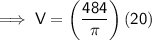
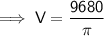
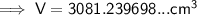
Therefore, the volume of the cylinder is 3081.24 cm³ (nearest hundredth).