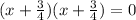Answer:
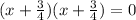
Explanation:
We are given the quadratic equation

Now, the roots of the quadratic equation
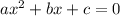 are given by
are given by
 .
.
So, from the given equation, we have,
a = 16, b =24 , c = 9.
Substituting the values in
 , we get,
, we get,
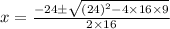
i.e.

i.e.
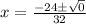
i.e.
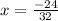
i.e.
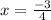
Thus, the roots of the equation are
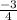 and
and
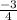 .
.
Hence, the factored form of the given expression will be