Answer: 32 inches
Explanation:
Since all the interior angles in a rectangle are right angles.
Therefore, the triangle which is formed by the diagonal and the shorter side of the tile with the longer side will be right triangle.
By trigonometry, in right triangle
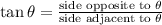
let x be the smaller side.
Therefore,
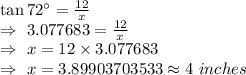
The perimeter of the rectangular tile=
