Answer:
Multiplication property of equality:
If a = b then,
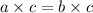
Addition property of equality:
If a = b then;

Subtraction property of equality:
If a = b then;
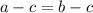
Division property of equality:
If a = b then;
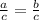
Substitution Property of equality:
if a = b then a can be substituted in b , and b can be substituted in a.
Given the system of equation:
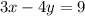 .....[1]
.....[1]
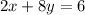 ......[2]
......[2]
Using multiplication property of equality
Multiply by 2 in equation [1] both sides we get;
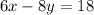 .....[3]
.....[3]
Using addition property of equality:
Add equation [2] and [3] to eliminate y we have;

Using Division property of equality:
Divide by 8 to both sides of an equation we have;
x = 3
Using substitution property of equality:
Substitute the value of x in equation [1] we have;
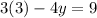
9-4y = 9
Using subtraction property of equality:
Subtract 9 from both sides we get;
-4y = 0
Divide by 4 to both sides we get;
y = 0