Question

A certificate of deposit earns 1% interest every three months. The interest is compounded.

What is the value of a $35,000 investment after 6 years?


$37,153.21

$39,438.88

$44,440.71

$56,295.30

Answer 1

A certificate of deposit earns 1% interest every three months. The interest is compounded.


What is the value of a $35,000 investment after 6 years?


$37,153.21

$39,438.88

$44,440.71

$56,295.30

A. 37,153.21

Answer 2

Option C.

Explanation:

The formula for amount after compound interest is

`A=P(1+(r)/(n))^(nt)`

where, P is principal, r is annual rate of interest, r/n is per period interest, n is number of time interest compounded in 1 year, t is number of years.

Given information:

Principle :
`P=35000`

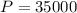
Per period interest :
`(r)/(n)=1\%=0.01`

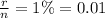
Number of time interest compounded in 1 year :
`n=4`

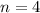
Number of years :
`t=6`

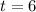
Using the formula we get

`A=35000\left(1+0.01\right)^(\left(4\cdot6\right))`

`A=35000\left(1.01\right)^(\left(24\right))`

`A=44440.7126986`

`A\approx 44440.71`

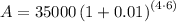
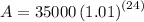
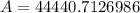
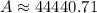
The value of a $35,000 investment after 6 years is $44,440.71.

Therefore, the correct option is C.