Answer: C.
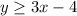
Explanation:
1. The graph indicates that the line intersect the y-axis at -4.
2. Then, you must choose any point that are located within the region and replace them to see if the inequality is satisfied. Therefore, you have:
Point (0,2):
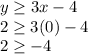
(This is true)
3. Therefore, you can conclude that the answer is the option C.