Question

How do you graph distance and time for an object that moves at a constant speed?

Answer 1

it is a diagonally straight line

Step-by-step explanation:

Answer 2

It would be a straight line

Step-by-step explanation:

On a distance-time graph, an object that moves at constant speed would be represented by a straight line.

In fact, in a distance-time graph, the slope of the line corresponds to the speed of the object. We can demonstrate that. In fact:

- The speed of the object is equal to the ratio between the distance covered
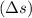`(\Delta s)` and the time taken (
`\Delta t`):

`v=(\Delta s)/(\Delta t)`

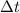On a distance-time graph, the distance is on the y-axis while the time is on the x-axis. The slope of the line is defined as:

`m=(\Delta y)/(\Delta x)`

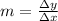
But the variation on the y-axis (
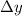`\Delta y`) is equal to the distance covered (
`\Delta s`), while the variation on the x-axis
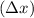`(\Delta x)` corresponds to the time taken (
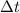`\Delta t`), so the slope can also be rewritten as

`m=(\Delta s)/(\Delta t)`

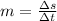
which is equal to the speed of the object. Therefore, an object moving at constant speed would be represented by a line with constant slope, which means a straight line.