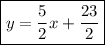Find the slope-intercept form of the equation of the line that passes through the point (5,1) and is perpendicular to the line 2x + 5y = 10

Question

asked Jul 25, 2020 41.7k views

1 Answer

Adrian Garner · Answer 1 · 2020-07-29T10:33:43+0000

The slope-intercept form:

y=mx+b

m - slope

b - y-intercept

Convert 2x + 5y = 10 to the slope0intercept form:

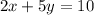2x+5y=10 subtract 2x from both sides

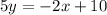5y=-2x+10 divide both sides by 5

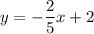
y=-(2)/(5)x+2

Let
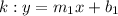k:y=m_1x+b_1 and
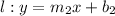
l:y=m_2x+b_2

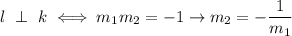
$l\ \perp\ k\iff m_1m_2=-1\to m_2=-(1)/(m_1)$

We have
m_1=-(2)/(5)

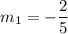
Therefore

$m_2=-\frac{-(2)/(5)}=\dfac{5}{2}$

We have the equation of a line:

y=(5)/(2)x+b

Put the coordinates of the point (5, 1) to the equation of a line:

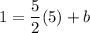
1=(5)/(2)(5)+b

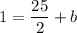1=(25)/(2)+b subtract
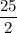from both sides

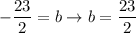
$-(23)/(2)=b\to b=(23)/(2)$

Answer:
$\boxed{y=(5)/(2)x+(23)/(2)}$