Question

State the order and type of each transformation of the graph of the function ƒ(x) = 4(5x)3 – 6 as compared to the graph of the base function

Answer 1

For the function,
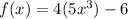`f(x) = 4(5x^3) -6`, stretches the graph by making the outputs matched to the inputs much larger than the parent function
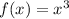`f(x) = x^3`. It stretches further by being multiplied by 4 after
`5^3=125`. Lastly the graph is shifted down by 6 units.

Explanation:

When functions are transformed there are a few simple rules:

• Adding/subtracting inside the parenthesis to the input shifts the function left(+) and right(-).
• Adding/subtracting outside the parenthesis to the output shifts the function up(+) and down(-).
• Multiplying the function by a number less than 1 compresses it towards the x-axis.
• Multiplying the function by a number greater than 1 stretches it away from the x-axis.
• Multiplying by a negative or changing the leading coefficient's sign will flip the graph.

For the function,
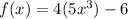`f(x) = 4(5x^3) -6`, stretches the graph by making the outputs matched to the inputs much larger than the parent function
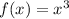`f(x) = x^3`. It stretches further by being multiplied by 4 after
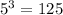`5^3=125`. Lastly the graph is shifted down by 6 units.