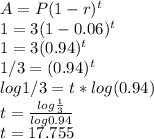Question

There is a drought and the oak tree population is decreasing at the rate of 6% per year. If the population continues to decrease at the same rate, how long will it take for the population to be a third of what it is? If necessary, round your answer to the nearest tenth. The population will reach a third of its original value in approximately years

Answer 1

17.8 years

Explanation:

To find the population in a future year, use the formula:

`A = P (1+/-r)^t`

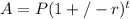
where A is the amount, p is the starting population, r is 6% or 0.06, and t is the number of years.

Since the population is decreasing it is subtraction. Here we are finding t, A=1 P =3, and r=0.06.

`A = P (1-r)^t\\1 = 3 (1-0.06)^t\\1=3(0.94)^t\\1/3=(0.94)^t\\log 1/3=t* log (0.94)\\t=\frac{log {(1)/(3)}}{log 0.94}\\t=17.755`