Answer:
Answer: Option d. (-63) is the sum of first 6 terms
Explanation:
Given sequence is 3-6+12-24+...........
We know the formula for the sum of the sequence be
S(6) = A(1)×

From the given question n= 6
A(1) = 3
and common ratio r = (-6)/3 = (-2)
Now we put these values in the formula
S(6) = 3×
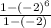
= 3×
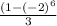
= 3×(1-64)/3
= 3×(-63)/3
= (-63)
So the sum of six terms is (-63).