Answer: The answer is
![x^2y^2\sqrt[3]{y^2}.](https://img.qammunity.org/2020/formulas/mathematics/middle-school/16w2s6i7dlavrdg0f2lbf30wiplv8esegt.png)
Explanation: The given expression is as follows
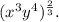
We are given to convert the above expression to the simplest radical form. For that, first we need to evaluate the n-th roots, then we need to evaluate the integral powers, and then we can reach at our desired result.
The conversion is as follows -
![(x^3y^4)^{(2)/(3)}\\\\=\sqrt[3]{(x^3y^4)^2}\\\\ =\sqrt[3]{x^6y^8}\\\\=x^{(6)/(3)}y^{(8)/(3)}\\\\=x^2y^{2+(2)/(3)}\\\\=x^2y^2y^(2)/(3)\\\\=x^2y^2\sqrt[3]{y^2}.](https://img.qammunity.org/2020/formulas/mathematics/middle-school/f9ckb52m0imt7lqu0kt27z27hkquzfr671.png)
Thus, the answer is
![x^2y^2\sqrt[3]{y^2}.](https://img.qammunity.org/2020/formulas/mathematics/middle-school/16w2s6i7dlavrdg0f2lbf30wiplv8esegt.png)