Question

Find the equation of the line that passes through the point of intersection of x + 2y = 9 and 4x -2y = -4 and the point of intersection of intersection of the lines 3x - 4y = 14 and 3x + 7y = -8.

Answer 1

`y=-6x+10`

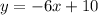
Explanation:

The point of intersection of

`x+2y=9...eqn1`

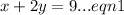
and

`4x-2y=-4...eqn2`

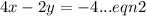
is the solution of the two equations.

We add equation (1) and equation(2) to get,

`x+4x+2y-2y=9+-4`

`\Rightarrow 5x=5`

`\Rightarrow x=1`

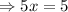
We put
`x=1` into equation (1) to get,

`1+2y=9`

`\Rightarrow 2y=9-1`

`\Rightarrow 2y=8`

`\Rightarrow y=4`

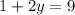
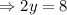
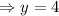
Therefore the line passes through the point,
`(1,4)`.

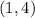The line also passes through the point of intersection of

`3x-4y=14...eqn(3)`

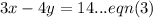
and

`3x+7y=-8...eqn(4)`

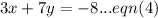
We subtract equation (3) from equation (4) to obtain,

`3x-3x+7y--4y=-8-14`

`\Rightarrow 11y=-22`

`\Rightarrow y=-2`

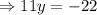
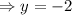
We substitute this value into equation (4) to get,

`3x+7(-2)=-8`

`3x-14=-8`

`3x=-8+14`

`3x=6`

`x=2`

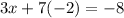
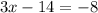
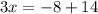
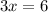
The line also passes through

`(2,-2)`

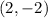
The slope of the line is

`slope=(4--2)/(1-2) =(6)/(-1)=-6`

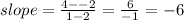
The equation of the line is

`y+2=-6(x-2)`

`y+2=-6x+12`

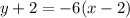
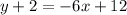
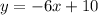`y=-6x+10` is the required equation