Answer:
9. B.
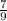
10. A.
Explanation:
9. We have been asked to find the quotient of expression
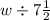 for
for
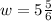 .
.
Let us substitute value of w in our division problem.
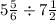
Let us convert our given mixed fractions into improper fractions.

Since we know that dividing a fraction by another fraction is same as multiplying the 1st fraction by the reciprocal of the second fraction. So flipping our second fraction and multiplying by 1st fraction we will get,

Upon canceling out greatest common factors we will get.
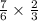
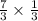
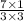
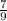
Therefore, our quotient will be
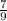 and option B is the correct choice.
and option B is the correct choice.
10. We are asked to find the product of
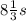 for
for
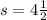 .
.
Upon substituting
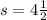 in our given expression we will get,
in our given expression we will get,

Let us convert our given mixed fractions into improper fractions.
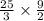
Upon canceling out greatest common factors we will get.
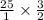
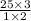
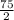
Upon converting our answer to mixed fraction we will get,
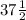
Therefore, product of our given problem will be
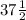 and option A is the correct choice.
and option A is the correct choice.