Question

What is the standard form of this complex number? ( 2 + 2i) / (1 - i)

Answer 1

`0+2i`

Explanation:

The given complex number is

`(2+2i)/(1-i) =(2(1+i))/(1-i)`

We multiply by the conjugate of the denominator to get,

`=(2(1+i)(1+i))/((1-i)(1+i))`

We apply difference of two squares and perfect squares to obtain,

`=(2(1+2i+i^2))/((1^2-i^2))`

`=(2(1+2i+-1))/((1--1))`

`=(2(1+2i-1))/((1+1))`

`=(2(2i))/((2))=2i`

In standard form we rewrite as
`a+bi`

The given complex number in standard form is

`0+2i`

Answer 2

To divide the complex number, we multiply top and bottom of the fraction by the conjugate of the denominator.

Given the complex number:
`(2+2i)/(1-i)`

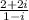
Multiply the conjugate of the denominator (1 + i) to the top and bottom.

we have;

`((2+2i) \cdot (1+i))/((1-i)\cdot (1+i))`

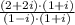
Using distributive property;
`a\cdot (b+c) = a\cdot b+ a\cdot c`

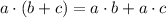
`(2+2i+2i+2i^2)/(1-i^2) = (2+4i-2)/(1-(-1)) = (0+4i)/(1+1) = (4i)/(2)= 2i` [ Since,
`i^2 = -1` ]

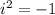Therefore, the standard form of this complex number
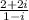`(2+2i)/(1-i)` is; 0 + 2i