Answer:
Explanation:
First we can determine the x value of our vertex via the equation:
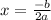
Note that in general a quadratic equation is such that:
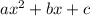
In this case a,b and c are the coefficients and so a=1, b=6 and c=13.
Therefore we can determine the x component of the vertex by plugging in the values known and so:
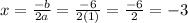
Now we can determine the y-component of our vertex by plugging in the x-component to the equation and so:
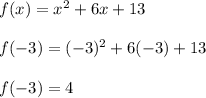
Therefore our vertex is (-3,4). Now in vertex our x component determines is the axis of symmetry so the equation for axis of symmetry is:
x=-3
Similarly, the y-component of our vertex is the minimum or maximum. In this case it is the minimum you can determine this because a is positive meaning that the parabola will point up, and so the equation for the minimum is:
y=4
The range of the formula is the smallest y-value meaning the minimum y=4 and all real numbers that are more than 4, mathematically:
Range = All real numbers greater than or equal to 4.