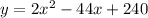Question

Find the equation of the quadratic function with zeros 10 and 12 and vertex at (11, -2).

Answer 1

`y=2x^2-44x+240`

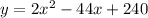
Explanation:

we are given a quadratic equation

Let's assume formula of vertex form of parabola as

`y=a(x-h)^2+k`

where vertex is (h,k)

we are given

vertex =(11,-2)

so, h=11, k=-2

now, we can plug it

`y=a(x-11)^2-2`

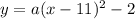
now, we are given zeros at x=10 and x=12

we know that zeros will be x=10 , y is 0

so, we can plug x=10 and y=0 and solve for 'a'

`0=a(10-11)^2-2`

`0=a-2`

`a=2`

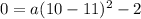
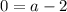
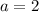
now, we can plug it back

and we get

`y=2(x-11)^2-2`

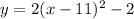
so, we get quadratic equation as

`y=2x^2-44x+240`