Please help! Find the ratio of the area of triangle XBY to the area of triangle ABC for the given measurements, if XY = 2, AC = 3 a. 1/3 b. 2/3 c. 4/9

Question

asked Sep 19, 2020 73.7k views

2 Answers

Yukari · Answer 1 · 2020-09-25T02:57:02+0000

Answer:

(c)

Explanation:

Consider ΔXBY and ΔABC

∠XYB = ∠ACB [ Corresponding angles of parallel line (AC and XY) are equal]

∠XBY = ∠ABC (Common angles)

By AA similarity, ΔXBY
$\sim$ ΔABC,

We know that the ratio of area of two similar triangles is the square of ratios of their sides.

Ratio of sides =
(XY)/(AC)

=
(2)/(3)

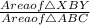$(Area of \triangle XBY)/(Area of \triangle ABC)$ =

=
[(3)/(2)]^(2)

=
(4)/(9)

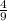
Option (c) is correct