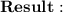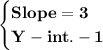SOLVING
If a linear function contains these points: (0,-1) and (3,8), what is its slope and y-int.?

Formula utilised, here
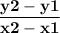 .
.
Put in the values,
 | subtract on top and bottom
| subtract on top and bottom
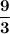 | divide on top and bottom
| divide on top and bottom
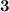
The y-intercept is the second co-ordinate of the point (0,-1)
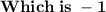 .
.