Answer:
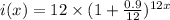 and growth rate factor is 0.075
and growth rate factor is 0.075
Explanation:
The function that models the population of iguanas in a reptile garden is given by
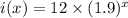 , where x is the number of years.
, where x is the number of years.
Since,
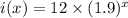
i.e.
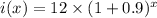 .
.
Therefore, the monthly growth rate function becomes,
i.e.
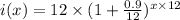 .
.
i.e.
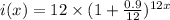 .
.
Hence, the monthly growth rate is i.e.
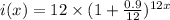 .
.
Also, the growth factor is given by
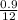 = 0.075.
= 0.075.
Thus, the growth factor to nearest thousandth place is 0.075.