Answer:
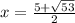 or
or
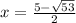
Explanation:
Given
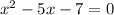
Required
Solve for x using:
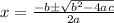
First, we need to identify a, b and c
The general form of a quadratic equation is:

So, by comparison with
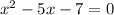
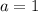
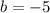
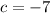
Substitute these values of a, b and c in
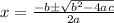

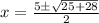
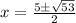
Split the expression to two
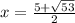 or
or
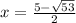
To solve further in decimal form, we have
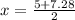 or
or
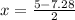
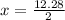 or
or

 or
or
