Answer:

Explanation:
The nth term of an arithmetic sequence can be found using the following formula.

Where n is the term, a₁ is the first term, and d is the common difference.
We want to find the 21st term, we know the first term is -6, and the common difference is 4.
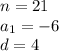
Substitute the values into the formula.
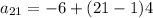
Solve according to PEMDAS: Parentheses, Exponents, Multiplication, Division, Addition, Subtraction.
Solve inside the parentheses.
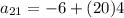
Multiply 20 and 4.
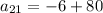
Add -6 and 80.
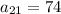
The 21st term of the sequence is 74