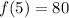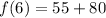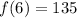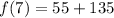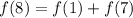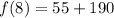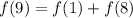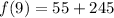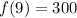Question

7. Are the ordered pairs below an arithmetic sequence or geometric

sequence?
(1,55)
(2, 45)
(3, 35)
(4, 25)


8. Using the formula: f(n) = f(1) + d(n-1), find f(9) for the ordered pairs
in #7.

Answer 1

8. Arithmetic Progression

9.
`f(9) = 300`

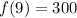
Explanation:

Given

`\{(1,55)\ (2, 45)\ (3, 35)\ (4, 25)\}`

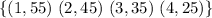
Solving (8): Arithmetic or Geometric

We start by checking if it is arithmetic by checking for common difference (d).

`d = y_2 - y_1 = y_3 - y_2 = y_4 - y_3`

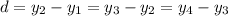
This gives:

`d = 45 - 55 = 35 - 45 = 25 - 35`

`d = -10 = -10 = -10`

`d=-10`

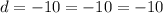
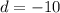
Because the common difference is equal, then it is an arithmetic progression

Solving (8):

`f(n) = f(1) + f(n-1)`

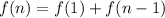
To find f(9), we substitute 9 for n

`f(9) = f(1) + f(9-1)`

`f(9) = f(1) + f(8)`

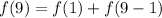
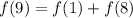
We need to solve for f(8); substitute 8 for n

`f(8) = f(1) + f(8 - 1)`

`f(8) = f(1) + f(7)`

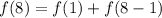
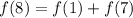
We need to solve for f(7); substitute 7 for n

`f(7) = f(1) + f(7 - 1)`

`f(7) = f(1) + f(6)`

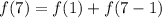
We need to solve for f(6); substitute 6 for n

`f(6) = f(1) + f(6 - 1)`

`f(6) = f(1) + f(5)`

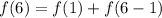
We need to solve for f(5); substitute 6 for n

`f(5) = f(1) + f(5 - 1)`

`f(5) = f(1) + f(4)`

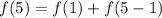
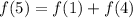
From the function, f(4) = 25 and f(1) = 55.

So:

`f(5)=55 + 25`

`f(5)=80`

`f(6) = f(1) + f(5)`

`f(6) = 55 + 80`

`f(6) = 135`

`f(7) = f(1) + f(6)`

`f(7) = 55 + 135`

`f(7) = 190`

`f(8) = f(1) + f(7)`

`f(8) = 55 + 190`

`f(8) = 245`

`f(9) = f(1) + f(8)`

`f(9) = 55 + 245`

`f(9) = 300`