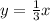Complete Question:
The given line segment has a midpoint at (3, 1). On a coordinate plane, a line goes through (2, 4), (3, 1), and (4, -2).
What is the equation, in slope-intercept form, of the perpendicular bisector of the given line segment?
Answer:
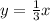
Explanation:
From the question, we understand that the line goes through
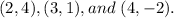
First, we calculate the slope of the above points

Where

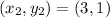
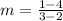


Also; from the question, we understand that the line segment is perpendicular to the above points.
This slope (m2) of the line segment is calculated as:
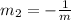
Substitute -3 for m

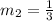
Lastly, we calculate the equation of the line using:
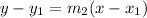
The line segment has a midpoint at (3, 1)
So:
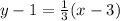
Open bracket
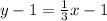
Add 1 to both sides

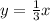
Hence, the equation of the line segment is: