Question

A pendulum on earth oscillates with a period of 3.45 seconds. What is the length of the pendulum?

Answer 1

The length of the pendulum is 2.954 m.

Step-by-step explanation:

Given;

period of the pendulum, T = 3.45 s

The period of the pendulum oscillation is given as;

`T = 2\pi \sqrt{(l)/(g) } \\\\(T)/(2\pi) = \sqrt{(l)/(g) }\\\\(T^2)/(4\pi ^2) = (l)/(g) \\\\l = (gT^2)/(4\pi ^2) \\\\`

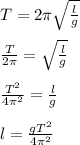
where;

L is length of the pendulum

g is acceleration due to gravity on Earth = 9.8 m/s²

`l = (gT^2)/(4\pi ^2)\\\\l = ((9.8)(3.45)^2)/(4\pi ^2)\\\\l = 2.954 \ m`

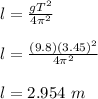
Therefore, the length of the pendulum is 2.954 m.