Note: Your question sounds a little unclear, but I am assuming that your system of equations is:


It would anyways clear your concept because the procedure to find the solutions remains the same for any set of a system of equations.
Answer:
The solution of the system of equations be:

Explanation:
Given the system of equations
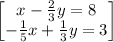
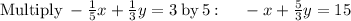
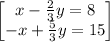
so adding the equation




so the system equations become
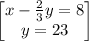
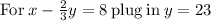
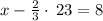
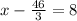
Add 46/3 to both sides
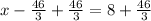
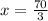
Therefore, the solution of the system of equations be:
