Question

If the length of a simple pendulum is increased by 4% and the mass is decreased by 4%, the period is:_________ A. not changed. B. increased by 2%. C. decreased by 4%. D. increased by 4%. E. decreased by 2%.

Answer 1

D i think, sorry if i got it wrong

Step-by-step explanation:

Answer 2

Answer B (Increased by 2%)

Step-by-step explanation:

Recall that the period (T) of a pendulum doesn't depend on the pendulum's mass, but depends on the pendulum's length (L) and on the local acceleration of gravity (g) via the formula:

`T=2\pi\,\sqrt{(L)/(g) }`

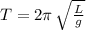
There fore, if the length of the pendulum is increased by 4% (0.04 in decimal form), then the new length becomes: L + 0.04 L = 1.04 L

and therefore the period will change by:

`T'=2\pi\,\sqrt{(1.04\,L)/(g) } = √(1.04) \,T\approx 1.02\,T`

Which means that the period was increased to about 2 % :

T + 0.02 T = 1.02 T