Question

A report states that the mean yearly salary offer for students graduating with a degree in accounting is $48,734. Suppose that a random sample of 50 accounting graduates at a large university who received job offers resulted in a mean offer of $49,830 and a standard deviation of $3600. Do the sample data provide strong support for the claim that the mean salary offer for accounting graduates of this university is higher than the national average of $48,734

Answer 1

To claim to be tested is whether "the mean salary is higher than 48,734".

i.e. μ > 48,734

Therefore the null and the alternative hypothesis are

`$H_0 : \mu = 48,734$`

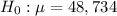
and
`$H_1 : \mu > 48,734$`

Here, n = 50

`$\bar x = 49,830$`

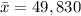
s = 3600

We take , α = 0.05

The test statistics t is given by

`$t=(\bar x - \mu)/((s)/(\sqrt n))$`

`$t=(49,830 - 48,734)/((3600)/(\sqrt 50))$`

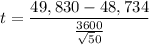
t = 2.15

Now the ">" sign in the
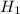`$H_1$` sign indicates that the right tailed test

Now degree of freedom, df = n - 1

= 50 - 1

= 49

Therefore, the p value = 0.02

The observed p value is less than α = 0.05, therefore we reject
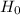`$H_0$`. Hence the mean salary that the accounting graduates are offered from the university is more than the average salary of 48,734 dollar.