Question

A company sells widgets. The amount of profit, y, made by

the company, is related to the selling price of each widget, x,
by the given equation. Using this equation, find out the
maximum amount of profit the company can make, to the
nearest dollar.
y = –2x2 + 105x – 773

Answer 1

Answer: 605

y=$605→Max profit

Answer 2

The maximum profit will be: $605.125

Explanation:

Given the function

`y\:=\:-2x^2\:+\:105x\:-\:773`

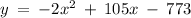
The given equation is a quadratic function. It represents Parabola. The parabola opens down because of the negative leading coefficient (-2).

Thus, the maximum profit would be computed at the vertex of the graph.

Thus, we have to determine the value of y when x is the line of symmetry.

We can find this by the equation

x = -b/2a

where a = -2, b = 105

x = -105 / 2(-2)

x = -105 / -4

x = 105/4

x = 26.25

Now, putting x = 26.25 in the original function to find the value of 'y'.

`y\:=\:-2x^2\:+\:105x\:-\:773`

`y=-2\left(26.25\right)^2+105\left(26.25\right)-773`

`y=-2\cdot \:26.25^2+105\cdot \:26.25-773`

`y=1983.25-1378.125`

`y=605.125`

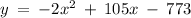
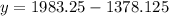
Therefore, the maximum profit will be: $605.125