Question

A (10.0+A) g ice cube at -15.0oC is placed in (125 B) g of water at 48.0oC. Find the final temperature of the system when equilibrium is reached.

Specific heat of ice: 2.090 J/g K
Specific heat of water: 4.186 J/gK
Latent heat of fusion for water: 333 J/g

Answer 1

Answer: Final temperature is 34.15°C.

Step-by-step explanation: When two objects have different temperature, they will exchange heat energy until there is no more net energy transfer between them. At that state, the objects are in thermal equilibrium.

So, when in equilibrium, the total heat flow must be zero, i.e.:

`Q_(1)+Q_(2)=0`

In our case, there will be a change in state of ice into water, so total heat flow will be:

`m_(1)c_(1)(T_(f)-T_(i))+m_(2)c_(2)(T_(f)-T_(i))+mL=0`

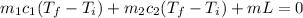
where

m₁ is mass of ice

m₂ is mass of water

c₁ is specific heat of ice

c₂ is specific heat of water

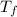`T_(f)` is final temperature

`T_(i)` is initial temperature

L is latent heat fusion

Temperature is in Kelvin so the transformation from Celsius to Kelvin:

For ice:

T = -15 + 273 = 258K

For water:

T = 48 + 273 = 321K

Solving:

`21(2.09)(T_(f)-258)+158(4.186)(T_(f)-321)+21(333)=0`

`43.89T_(f)-11323.62+661.4T_(f)-212305.55+6993=0`

`705.3T_(f)=216636.17`

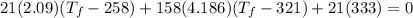
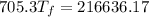
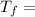`T_(f)=` 307.15K

In Celsius:

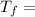`T_(f)=` 34.15°C

Final temperature of the system when in equilibrium is 34.15°C