Question

Complete the square to make a perfect square trinomial. Then, write the result as a binomial squared. x2+3/2x

Answer 1

The added term needed for that expression to be a square is 9/16.

Explanation:

We're provided with two terms, and asked to add an additional term to make this a perfect square. For this to work, the term needs to be a scalar value that is the square of half the coefficient of the second term.

That coefficient is 3/2, so half of that is 3/4, and its square is 9/16.

If we tack that on the end then, we get:

`x^2 + (3x)/(2) + (9)/(16)\\=(x + (3)/(4))^2`

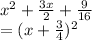
To confirm the answer, let's expand it and see if we get the original expression:

`(x + (3)/(4))^2\\= (x + (3)/(4))(x + (3)/(4))\\= x^1 + (3x)/(4) + (3x)/(4) + (9)/(16)\\= x^2 + (6x)/(4) + (9)/(16)\\=x^2 + (3x)/(2) + (9)/(16)`

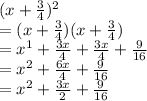
So 9/16ths is the scalar that needs to be added on the end.