Question

What is the average rate of change of f over the interval -7 < or equal to x Give an exact number

Answer 1

The average rate of change of f over the interval -7 < x is calculated by finding the difference in the y-values of f and dividing it by the difference in the x-values over that interval.

Step-by-step explanation:

The average rate of change of f over the interval -7 < x is the change in the y-values of f divided by the change in the x-values over that interval. To find the average rate of change, we need to evaluate f(x) at the endpoints of the interval and calculate the difference in the y-values. Then we divide that difference by the difference in the x-values.

For example, if f(x) = x2 and we have the interval -7 < x < 3, the average rate of change of f over that interval would be:

Average rate of change = (f(3) - f(-7)) / (3 - (-7)) = (9 - 49) / (3 + 7) = -40 / 10 = -4.

Answer 2

`(4)/(3)`

Step-by-step explanation:

The average rate of change can be found by using the following formula

`(f(x_(2)) - f(x_(1)) )/(x_(2) - x_(1))`

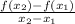
Since the interval goes from -7 to 2, we should find the y-values that correspond to x=-7 and x=2.

By inspection of the graph, we can clearly see that when x is -7, y is -7 as well and when x is 2, y is 5.

Now that we know this, we can simply plug these values into the formula!

`(f(2) - f(-7) )/(2 - (-7)) = (5 - (-7))/(2 - (-7)) = (5 +7)/(2+7) = (12)/(9) = (4)/(3)`

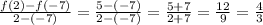
Good luck!