Question

`\underline{ \underline{ \text{question}}} :` Find the equation of straight line which cuts off an intercept 2 from the Y - axis and whose perpendicular distance from the origin is 1.

​

Answer 1

the answer for this question is the picture hope this helps

Answer 2

`y=-√(3)x+2`

Explanation:

We want to find the equation of a straight line that cuts off an intercept of two from the y-axis and whose perpendicular distance from the origin is one.

Let Point M be (x, y). As we know, Point R will be (0, 2) and Point O (the origin) will be (0, 0).

First, we can use the distance formula to determine values for M. The distance formula is given by:

`\displaystyle d=√((x_2-x_1)^2+(y_2-y_1)^2)`

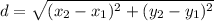
Since we know that the distance between O and M is one, d = 1:

And we will let M(x, y) be (x₂, y₂) and O(0, 0) be (x₁, y₁). So:

`\displaystyle 1=√((x-0)^2+(y-0)^2)`

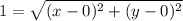
Simplify:

`1=√(x^2+y^2)`

We can solve for y. Square both sides:

`1=x^2+y^2`

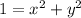
Rearranging gives:

`y^2=1-x^2`

Take the square root of both sides. Since M is in the first quadrant, we only need to worry about the positive case. Therefore:

`y=√(1-x^2)`

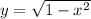
So, Point M is now given by (we substitute the above equation for y):

`M(x,√(1-x^2))`

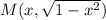
We know that Segment OM is perpendicular to Line RM.

Therefore, their slopes will be negative reciprocals of each other.

So, let’s find the slope of each segment/line. We will use the slope formula given by:

`\displaystyle m=(y_2-y_1)/(x_2-x_1)`

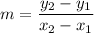
Segment OM:

For OM, we have two points: O(0, 0) and M(x, √(1-x²)). So, the slope will be:

`\displaystyle m_(OM)=(√(1-x^2)-0)/(x-0)=(√(1-x^2))/(x)`

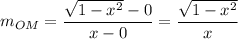
Line RM:

For RM, we have the two points R(0, 2) and M(x, √(1-x²)). So, the slope will be:

`\displaystyle m_(RM)=(√(1-x^2)-2)/(x-0)=(√(1-x^2)-2)/(x)`

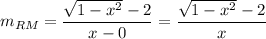
Since their slopes are negative reciprocals of each other, this means that:

`m_(OM)=-(m_(RM))^(-1)`

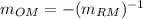
Substitute:

`\displaystyle (√(1-x^2))/(x)=-\Big((√(1-x^2)-2)/(x)\Big)^(-1)`

Now, we can solve for x. Simplify:

`\displaystyle (√(1-x^2))/(x)=(x)/(2-√(1-x^2))`

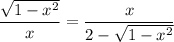
Cross-multiply:

`x(x)=√(1-x^2)(2-√(1-x^2))`

Distribute:

`x^2=2√(1-x^2)-(√(1-x^2))^2`

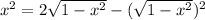
Simplify:

`x^2=2√(1-x^2)-(1-x^2)`

Distribute:

`x^2=2√(1-x^2)-1+x^2`

So:

`0=2√(1-x^2)-1`

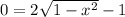
Adding 1 and then dividing by 2 yields:

`\displaystyle (1)/(2)=√(1-x^2)`

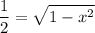
Then:

`\displaystyle (1)/(4)=1-x^2`

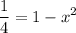
Therefore, the value of x is:

`\displaystyle \begin{aligned}(1)/(4)-1&=-x^2\\-(3)/(4)&=-x^2\\ (3)/(4)&=x^2\\ (√(3))/(2)&=x\end{aligned}`

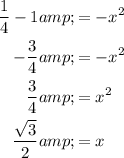
Then, Point M will be:

`\begin{aligned} \displaystyle M(x,√(1-x^2))&=M\left((√(3))/(2), \sqrt{1-\left((√(3))/(2)\right)^2}\right)\\ \\ M&=\left((\sqrt3)/(2),(1)/(2)\right)\end{aligned}`

Therefore, the slope of Line RM will be:

`\displaystyle \begin{aligned}m_(RM)&=((1)/(2)-2)/((√(3))/(2)-0) \\ \\ &=((-3)/(2))/((√(3))/(2))\\ \\ &=-(3)/(\sqrt3)\\ \\&=-\sqrt3\end{aligned}`

And since we know that R is (0, 2), R is the y-intercept of RM. Then, using the slope-intercept form:

`y=mx+b`

We can see that the equation of Line RM is:

`y=-√(3)x+2`