Question

how many different combinations of six people can sit in four chairs? assume one person sits in each chair

Answer 1

We want to choose 4 people and we have 6 people to choose from. This is a combination of 6, 4 by 4. Also called six choose four.

The following formula:

`_rC_n=(n!)/(r!(n-r)!)`

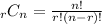
Is the combination of
`r` objects choosen from a total of
`n`. It's
`n` choose
`r`.

For our problem, we just need to compute the following:

`_4C_6=(6!)/(4!(6-4)!)=(6*5*4!)/(4!2!) =(6*5)/(2*1) =15`

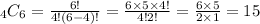
Thus

`\boxed{_4C_6=15}`

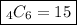
Therefore the answer is 15 different combinations