Question

Mose decides to launch a rocket out of the barn at Schrute farms. The equation below represents the height (in meters), of the rocket getting launched above the ground, t seconds after the rocket is launched. h(t) = -.4 (t - 2)^2 + 5 How high above the ground is the rocket before it is launched?

Answer 1

Height at an instance of time is given by :

h(t) = -4( t - 2 )² + 5 .....1

Differentiating above equation with respect to t .

`(dh)/(dt)= -4* ( -2 ) ( t - 2 ) \\\\(dh)/(dt)= 8(t-2)`

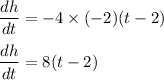
Now, for maximum ,
`(dh)/(dt) = 0` .

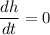8( t - 2 ) = 0

t = 2

Putting value of t = 2 in given equation, we get :

h( 2 ) = -4( 2 - 2 )² + 5

h( 2 ) = 5 m

Therefore, maximum height rocket can reach is 5 m.