Question

The company plans to have the tanks painted after they are installed. Describe the effect that doubling the diameter has on the amount of paint needed to paint the tanks. What happens when the diameter is increased by a factor of 3?

Answer 1

The effect of doubling the diameter is increased the area to paint as 4 times. So, paint needed is 4 times than it was before. And the area is increased by 9 times when the diameter is increased by a factor of 3. So, paint needed is 9 times than before.

Explanation:

Please remember the concept

If side lengths are in the ratio a : b

Then the area are in the ratio of a^2 :b^2

The volume are in the ratio of a^3 :b^3,

When diameter is doubled, the area ratio becomes
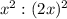`x^(2) :(2x)^(2)` which is simplified to
`x^(2) :4x^(2)`

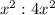
So, the area is increased by 4 times.

According to this concept , the diameter is increased by the scale factor 3.

Let the diameter of tank is 'x', so the diameter becomes "3x"

So, it's area ratio would be
`x^(2) :(3x)^(2)`

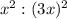
If we simplify it we get
`x^(2) :9x^(2)`

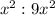
We conclude that area to be increased by 9 times.