Question

A gas sample occupies 4.39 L at 44 ºC. What will be the new volume, using Charles’ Law, if the temperature decreases to 25 ºC?

Answer 1

Hi there! :)

`\large\boxed{x = 2.49 L}`

Use the proportion for Charles' Law where:

`(v_(1))/(t_(1))= (v_(2))/(t_(2))`

v1 = initial volume

t1 = initial temperature

v2 = final volume

v2 = final temperature

Substitute in the given values into the proportion:

v1 = 4.39 L

t1 = 44° C

t2 = 25°C

v2 = x L

Set up the proportion:

`(4.39)/(44) = (x)/(25)`

Cross multiply:

`25 * 4.39 = 44x\\\\109.75 = 44x\\\\x = 2.49 L`

Answer 2

The new volume will be approximately 2.49 L.

Step-by-step explanation:

We are given that a gas sample occupies 4.39 L at 44° C.

We are also given that we are going decrease the temperature to 25° C.

Charles' Law has a formula which shows the relationship between the volumes of a samples and the temperatures of the samples.

The proportion is shown as:

`\displaystyle \bullet \ \ \ (V_1)/(T_1) = (V_2)/(T_2)`,

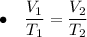where V₁ and V₂ are the initial and final volume respectively and T₁ and T₂ are the initial and final temperature respectively.

This proportion can be used to determine what our unknown is. We need to know the final volume.

`\displaystyle \frac{4.39 \ \text{L}}{44 \ \text{C}} = \frac{\text{x}}{25 \ \text{C}}\\\\\\(4.39)/(44) = (x)/(25)\\\\\\4.39 * 25 = 44 * x\\\\\\44x = 109.75\\\\\\(44x)/(44)=(109.75)/(44)\\\\\\x = 2.49432 \approx \boxed{2.49 \ \text{L}}`

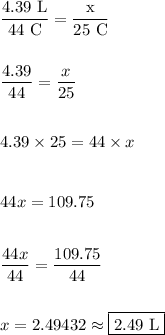
Therefore, if we reduce the temperature to 25 °C, we will be reducing the volume of the substance to 2.49 L.