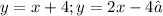Question

Y=-x-4

y=2x-2

y=-x+4
y=2x-4

y=x-4
y=2x-2

y=x+4
y=2x-4​

Answer 1

The slope of both the lines are
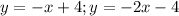`y=-x+4; y=-2x-4`. Therefore, the correct option is B.

Line 1 (Passing through points
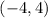`$(-4,4)$` and
`$(-1,-2)$`:

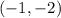1. Calculate the slope (
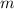`$m$` ) using the formula:
`$m=(y_2-y_1)/(x_2-x_1)$`

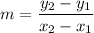
2. Substitute the coordinates of the two points into the formula:
`$m=(-2-4)/(-1-(-4))$`

3. Simplify the calculation:

`m=(-6)/(3) \\\ m = -2`

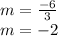
4. Use the point-slope form of the equation to get the equation of the line:

`$y-y_1=m\left(x-x_1\right)$`

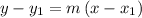
5. Choose one of the points for the equation, for example
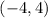`$(-4,4)$`, and substitute the values:

`y-4=-2(x+4) \\y-4=-2x-8 \\y=-2x-4`

Line 2 (Passing through points
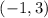`$(-1,3)$` and
`$(1,5)$`:

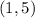1. Calculate the slope
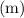`$(\mathrm{m})$` using the same formula as above.

2. Substitute the coordinates of the two points into the formula:
`$m=(5-3)/(1-(-1))$`

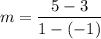
3. Simplify the calculation:

`$m=(2)/(2)$`

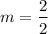
4. Simplify further to find the slope:
`$m=1$`

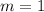
5. Use the point-slope form of the equation with one of the points, say
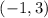`$(-1,3)$`, and substitute the values:
`$y-3=1(x+1)$`

6. Distribute and rearrange to get the standard form or slope-intercept form:
`y=x+4`

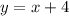
The correct options in the question are:

A.
`y=-x-4; y=2x-2`

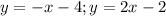
B.
`y=-x+4; y=-2x-4`

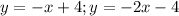
C.
`y=x-4; y=2x-2`

D.
`y=x+4; y=2x-4​`

Answer 2

Explanation:

I'm not sure if the answers you gave are incorrect, but the correct answer is:

y = x + 4

y = -2x - 4