Question

A person walks into a refrigerated warehouse with head uncovered. Model the head as a 25- cm diameter sphere at 35°C with a surface emissivity of 0.95. Heat is lost from the head to the surrounding air at 25°C by convection with a convection coefficient of ???????????????? ???????? ????????????????∙???????? , and by radiation to the surrounding black walls at 15°C. Determine the total rate of heat loss. StefanBoltzmann Constant, ???????? = ????????. ???????????????? × ????????????????−???????? ???????? ????????????????∙???????????????? . (10 points)

Answer 1

Hello some parts of your question is missing below is the missing part

Convection coefficient = 11 w/m^2. °c

answer : 44.83 watts

Step-by-step explanation:

Given data :

surface emissivity ( ε )= 0.95

head ( sphere) diameter( D ) = 0.25 m

Temperature of sphere( T ) = 35° C

Temperature of surrounding ( T∞ ) = 25°C

Temperature of surrounding surface ( Ts ) = 15°C

б = ( 5.67 * 10^-8 )

Determine the total rate of heat loss

First we calculate the surface area of the sphere

As =
`\pi D^(2)`

=
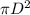
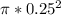`\pi * 0.25^2` = 0.2 m^2

next we calculate heat loss due to radiation

Qrad = ε * б * As(
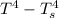`T^(4) - T^(4) _(s)` ) ---- ( 1 )

where ;

ε = 0.95

б = ( 5.67 * 10^-8 )

As = 0.2 m^2

T = 35 + 273 = 308 k

Ts = 15 + 273 = 288 k

input values into equation 1

Qrad = 0.95 * ( 5.67 * 10^-8 ) * 0.2 ( (308)^4 - ( 288)^4 )

= 22.83 watts

Qrad ( heat loss due to radiation ) = 22.83 watts

calculate the heat loss due to convection

Qconv = h* As ( ΔT )

= 11*0.2 ( 35 -25 ) = 22 watts

Hence total rate of heat loss

= 22 + 22.83

= 44.83 watts