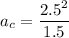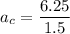Question

A ball is tied to a string and swung in a horizontal circle with a tangential speed of 2.5 m/s. The length of the string is 1.5m. Calculate the ball’s centripetal acceleration.

Answer 1

`a_c=4.17\ m/s^2`

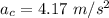
Explanation:

Centripetal Acceleration

It's the acceleration of an object moving in a uniform circular motion that pushed the object toward the center.

Its magnitude can be calculated as follows:

`\displaystyle a_c=(v^2)/(r)`

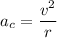
Where:

v = tangential speed

r = radius of the circle

The ball is tied to a string describing a circular motion with a radius of r=1.5 m. The tangential speed is v=2.5 m/s, thus the acceleration is:

`\displaystyle a_c=(2.5^2)/(1.5)`

`\displaystyle a_c=(6.25)/(1.5)`

`\mathbf{a_c=4.17\ m/s^2}`