Given:
The length of a rectangle is one unit more than its width.
Area of rectangle = 56 units
To find:
The dimensions of the rectangle.
Solution:
Let, width of the rectangle be x.
Then, length of the rectangle = x+1
Area of a rectangle is
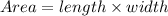
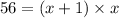
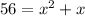
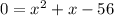
By splitting the middle term, we get
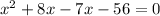

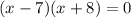
Using zero product property, we get
 and
and
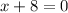
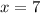 and
and

Width cannot be negative. So, x=7.
Now,
Width = 7 units
Length = 7+1
= 8 units
Therefore, the length of the rectangle is 8 units and width is 7 units.