Question

Please find the area of the given picture. Please as fast as possible........​

Answer 1

Area = 168 cm²

Explanation:

**Please note that there is an error in the drawing of the given parallelogram. In order for the parallelogram to have the given shape, the diagonal AC is actually 22.5 cm in length, whereas diagonal DB is 15 cm in length (see attached diagram). Therefore, I shall be using the attached diagram for my calculations. However, please note that due of the properties of a parallelogram, the final answer will be the same, regardless of which diagonal is used.**

To calculate the area of a parallelogram, we would usually multiply the base by the perpendicular height. As the perpendicular height is unknown in the given parallelogram, use the following formula:

`\textsf{Area of parallelogram} = ab \sin (x)`

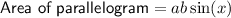
where:

• a and b are the lengths of parallel sides
• x is the included angle

As the diagonal of the parallelogram is given, use the cosine rule to calculate the measure of the included angle ∠DCB.

Cosine Rule (for finding angles)

`\sf \cos(C)=(a^2+b^2-c^2)/(2ab)`

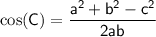
where:

• C is the angle
• a and b are the sides adjacent the angle
• c is the side opposite the angle

From inspection of the given diagram:

• C = ∠DCB
• a = DC = 13 cm
• b = CD = 14 cm
• c = DB = 15 cm

Substitute the given values into the cosine rule formula and solve for ∠ABC:

`\implies \sf \cos(DCB)=(13^2+14^2-15^2)/(2(13)(14))`

`\implies \sf \cos(DCB)=(140)/(364)`

`\implies \sf \cos(DCB)=(5)/(13)`

`\implies \sf \angle DCB=67.38013505...^(\circ)`

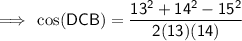
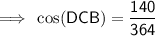
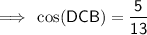
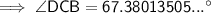
Substitute the found angle and the length of the parallel sides into the area formula:

`\begin{aligned}\textsf{Area of parallelogram} & = ab \sin (x)\\\\\implies \textsf{Area} & = \sf (13)(14) \sin (DCB)\\& = \sf (13)(14) \left((12)/(13)\right)\\& = \sf 168\:\:cm^2\end{aligned}`

Therefore, the area of the given parallelogram is 168 cm².