Answer:
Approximately
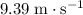 after the sphere has travelled a distance of
after the sphere has travelled a distance of
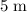 .
.
Approximately
 right before touching the ground (a distance of
right before touching the ground (a distance of
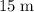 .)
.)
Assumption:
 .
.
Step-by-step explanation:
Weight of the sphere:
 , downwards.
, downwards.
Drag on the sphere:
 upwards.
upwards.
Net force on the sphere:
 downwards.
downwards.
Acceleration of the sphere:
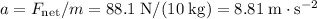 .
.
Apply the SUVAT equation
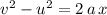 , where
, where
 is the final velocity,
is the final velocity,
 is the initial velocity (
is the initial velocity (
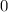 in this case, as the sphere was released from rest,) and
in this case, as the sphere was released from rest,) and
 is the distance (displacement) that the sphere has travelled so far.
is the distance (displacement) that the sphere has travelled so far.
Rearrange this equation to obtain an expression for
 :
:
 .
.
For example, after the ball travelled a distance of
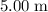 ,
,
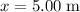 :
:
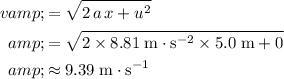 .
.
Similarly,
 right before landing, such that:
right before landing, such that:
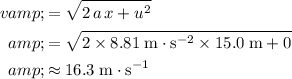 .
.