Question

G is the centroid of tiangle ABC, AB = AC, AD= 15, CG= 13, and AD is perpendicular to CB. Find the length of the segment. ​

Answer 1

AG=13

AB=AC

AD is | to BC

CG=BG

line segments is BG or GB

Answer 2

Since G is the centroid of triangle ABC, and AD is perpendicular to CB, the length of the segment include;

1. AG = 10 units.

2. GD = 5 units.

3. CD = 12 units.

4. GE = 6.5 units.

5. GB = 13 units.

In Mathematics and Geometry, the centroid theorem states that the centroid of a triangle is located at two-third (2/3) of the distance from the vertex to the midpoint of the (opposite) sides.

Part 1.

Based on the centroid theorem, we can logically deduce that point G represents the centroid of triangle ABC. If the length of AD is 15 units, the length of AG can be calculated as follows;

AG = 2/3 × AD

AG = 2/3 × 15

AG = 30/3

AG = 10 units.

Part 2.

For the length of GD, we have:

GD = AD - AG

GD = 15 - 10

GD = 5 units.

Part 3.

In order to determine the length of CD, we would apply Pythagorean's theorem;

`CD^2=CG^2-GD^2\\\\CD^2=13^2-5^2\\\\CD=√(169-25)`

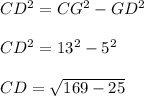
CD = 12 units.

Part 4.

If the length of CG is 13 units, the length of CE can be calculated as follows;

CG = 2/3 × CE

13 = 2/3 × CE

CE = 39/2

CE = 19.5 units.

Therefore, the length of GE is given by;

GE = CE - CG

GE = 19.5 - 13

GE = 6.5 units.

Part 5.

Based on the definition of a centroid, we can logically deduce the following congruent sides;

CG ≅ GB

CG = GB = 13 units.